This is one of my favorite derivations. Although it would sound a bit intimidating at first, as none of the standard textbooks carry out the derivation in curvilinear coordinates; it is rather easy to obtain. And guess what? the math is quite rewarding!
So we first have to start by selecting a convenient control volume. The idea here is to pick a volume whose sides are parallel per say to the coordinates. For cylindrical coordinates, one may choose the following control volume

Again, as we did in the previous post, we need to account for all the fluid that is accumulating, and flowing through this control volume, namely:
Rate of Rate of Flow In = Accumulation + Rate of Flow Out
or
Accumulation + Flow Out - Flow In = 0
First, let’s get some basics laid out. The velocity field will be described as

I always prefer to use u, v, and w instead of ur, utheta, and uz to save on subscripts, although the latter nomenclature is a bit more descriptive… we’ll get used to it. Now, by construction, the volume of the differential control volume is
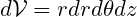
while the mass of fluid in the control volume is
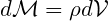
The rate of change of mass or accumulation in the control volume is then

For the net flow through the control volume, we deal with it one face at a time. Starting with the r faces, the net inflow is
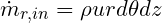
while the outflow in the r direction is
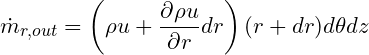
So that the net flow in the r direction is

Being O(dr^2), the last term in this equation can be dropped so that the net flow on the r faces is
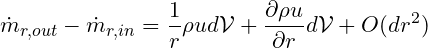
The net flow in the theta direction is slightly easier to compute since the areas of the inflow and outflow faces are the same. At the outset, the net flow in the theta direction is
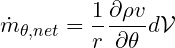
We now turn our attention to the z direction. The face area is that of a sector of angle d\theta:
then, the inflow at the lower z face is

while the outflow at the upper z face is
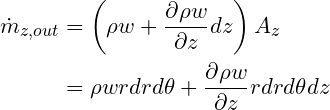
Finally, the net flow in the z direction is

Now we can put things together to obtain the continuity equation
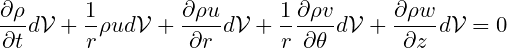
dividing by dV and rearranging the r components of the velocity
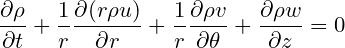
Voila!
[Previous: Continuity Eq. in Cartesian Coordinates]
So we first have to start by selecting a convenient control volume. The idea here is to pick a volume whose sides are parallel per say to the coordinates. For cylindrical coordinates, one may choose the following control volume

Again, as we did in the previous post, we need to account for all the fluid that is accumulating, and flowing through this control volume, namely:
Rate of Rate of Flow In = Accumulation + Rate of Flow Out
or
Accumulation + Flow Out - Flow In = 0
First, let’s get some basics laid out. The velocity field will be described as
I always prefer to use u, v, and w instead of ur, utheta, and uz to save on subscripts, although the latter nomenclature is a bit more descriptive… we’ll get used to it. Now, by construction, the volume of the differential control volume is
while the mass of fluid in the control volume is
The rate of change of mass or accumulation in the control volume is then
For the net flow through the control volume, we deal with it one face at a time. Starting with the r faces, the net inflow is
while the outflow in the r direction is
So that the net flow in the r direction is
Being O(dr^2), the last term in this equation can be dropped so that the net flow on the r faces is
The net flow in the theta direction is slightly easier to compute since the areas of the inflow and outflow faces are the same. At the outset, the net flow in the theta direction is
We now turn our attention to the z direction. The face area is that of a sector of angle d\theta:
then, the inflow at the lower z face is
while the outflow at the upper z face is
Finally, the net flow in the z direction is
Now we can put things together to obtain the continuity equation
dividing by dV and rearranging the r components of the velocity
Voila!
[Previous: Continuity Eq. in Cartesian Coordinates]
Cite as:
Saad, T. "8. Derivation of the Continuity Equation in Cylindrical Coordinates".
Weblog entry from
Please Make A Note.
http://pleasemakeanote.blogspot.com/2009/02/8-derivation-of-continuity-equation-in.html
Hi
ReplyDeleteI really need this equation & I dont know for what reason your blog is not working , is there anyway you can fix it or send it to me ????
Thanks buddy..i have been looking for these derivations in many texts...i used to get intimidated when i see the question in my exam..thank you..
ReplyDeletethanks a lot.
ReplyDeleteGood and very descriptive derivation. Is there any chance you've ever worked through this derivation in spherical coordinates? I'm slogging through it now, and I think I must be getting it wrong because I'm coming up with some stupidly complicated equations. If you've worked through it already, I'd appreciate any help you could offer. Email me at kbranha AT yahoo DOT com if you can help. Thanks.
ReplyDeleteThanks a lot! I have a question that why O(dr^2) can be dropped.
ReplyDeleteKen, I posted the derivation of the continuity equation in Spherical coordinates. You can find it here: http://pleasemakeanote.blogspot.com/2010/02/9-derivation-of-continuity-equation-in.html
ReplyDeleteOrion, thanks a lot for your post.
ReplyDeleteAs you know, dr, d\theta, and dz are all differential increments. Derivations that usually include such increments are valid in the limit as these increments approach zero. To be rigorous, one must carry out all these terms to the end. Those that do not cancel out will simply vanish in the limit. In this case, we know ahead of time that the higher order terms will vanish, so for simplicity, we don't carry them in the first place. Hope that clarifies things.
Thanks for work you are doing.
ReplyDeletewhy does (1/r)*p*u+(dpu/dt)=(1/r)(drpu/dt) in the last line? thanks
ReplyDeleteI think that you are referring to the d/dr term not the d/dt term. If so, then expand the following:
ReplyDelete(1/r)(drpu/dr) = (1/r) pu dr/dr + (1/r) r d(pu)/dr = (1/r) pu + d(pu)/dr
This was a very thorough derivation...I have about 5 texts on differential calculus and fluid mechanics and only one carries out any fundamental derivations that are not in cartesian coordinates (Bird Stewart and Lightfoot Transport Phenomena). Do you know of any solid texts that are thorough in this regard?
ReplyDeleteI have not seen this derivation in a reference system other than Cartesian coordinates. Thanks for the reference though.
ReplyDeleteThanks for your work.Could you guide me on how to get the equation when body forces are considered.
ReplyDeleteYou are AWESOME!!
ReplyDeleteI think there is a problem in your derivation.When you calculated the surface area in Z direction, you took dr as the height. Since you took dr equals to the edge, then that would be a regtangular but not a trapezoid. That is, given dr is the height, the way to calculate the area is dr*r*dtheta but not the way you present here although the results are the same.
ReplyDeleteThe height of the trapezoid is dr*cos(dtheta)~dr with vanishing dtheta. Therefore, with vanishing dtheta, the height of the trapezoid becomes dr.
ReplyDeleteIn your calculation A = dr*r*dtheta, you are assuming that the sector is a rectangle. This is only correct to second order. The area of that sector is:
A = (1/2)*(r+dr)^2*dtheta - (1/2)*r^2*dtheta
= r*dr*dtheta + (1/2)*dr^2*dtheta
which is exactely the area calculated by assuming that the sector is a trapezoid with height dr. To avoid any confusion, I've recalculated that area as a sector.
isn't mass balance " in - out = accumulation " . Why is it that you have taken it as out - in in all the directions?
ReplyDeleteYou are correct and this is exactly what I've done. If you notice, the resulting equation is:
DeleteAccumulation + Out - In = 0.
You may have been mislead by a typo that I had in the first paragraph but have since fixed.
Thanks dude...I m looking for this from many dates....its is really good...thanks
ReplyDeleteThanks really helped me with my engineering exams!
ReplyDeleteI am totally impressed. Keep up the good work.
ReplyDeleteExceptional talent you have got
Can u jus elaborate the derivation of net flow in theta direction?
ReplyDeleteThanks for sharing...
ReplyDeleteBest Wishes (:
Armin
thanks
ReplyDeletei want mathematical modelling of condensation inside horizontal tube but i am not able to get it will yo please help me
I've done this derivation using an element centered on radial coordinate r so that inner cylindrical face is at (r-dr/2) and outer face is at (r + dr/2). Follow same procedure, get same result, but the O(dr)^2 does not appear.
ReplyDeleteThanks a lot for the derivation. It is great that you are deriving something that most textbooks do not derive.
ReplyDeleteCan someone please explain the O(dr^2)?
ReplyDeleteHi, can i know where you got this question from
ReplyDeleteseems like a very good work but I don't know why I can't view the main pictures showing the actual derivation
ReplyDelete